State and Prove Gauss Theorem important topic

• Home
• Physics
• Electrostatics
• Gauss’s Theorem

State and Prove Gauss Theorem

Gauss’s Theorem

Gauss’s theorem is a fundamental principle in electrostatics that relates the electric field to the distribution of electric charges. It is also known as Gauss’s law.

It states that the total electric flux through any closed surface is equal to 1/ϵ₀ times the net electric charge enclosed by that surface.

Mathematically, Gauss’s theorem can be written as:

Φ = (Q_enclosed) / ϵ₀

Since electric flux can be represented in terms of electric field vector E and the area vector dS. So, above law can be written as,

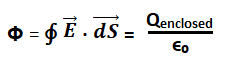
Φ = ∮E · dS cosθ = (Q_enclosed) / ϵ₀

where:

• ∮E · dS represents the closed surface integral of the dot product of the electric field vector E and the differential area vector dS.
• Q_enclosed is the net electric charge enclosed by the closed surface or Gaussian surface.
• ϵ₀ (epsilon naught) is the permittivity of free space.

Gauss’s theorem is used to easily calculate the electric field for symmetric charge distributions in comparison with Coulomb’s law. It simplifies the calculations by allowing the use of symmetry and providing a direct relationship between the electric field and the enclosed charge.

Important implications of Gauss’s theorem. If the net charge enclosed by a closed surface is zero (Q_enclosed = 0), Gauss’s theorem states that the total electric flux passing through the surface is also zero (∮E · dS = 0).

The Proof of Gauss’s Theorem

To prove Gauss’s Theorem in electrostatics, we will consider a closed surface S enclosing a charge distribution. The charge distribution may consist of multiple charges, but for the purpose of this proof, we will focus on a single point charge.

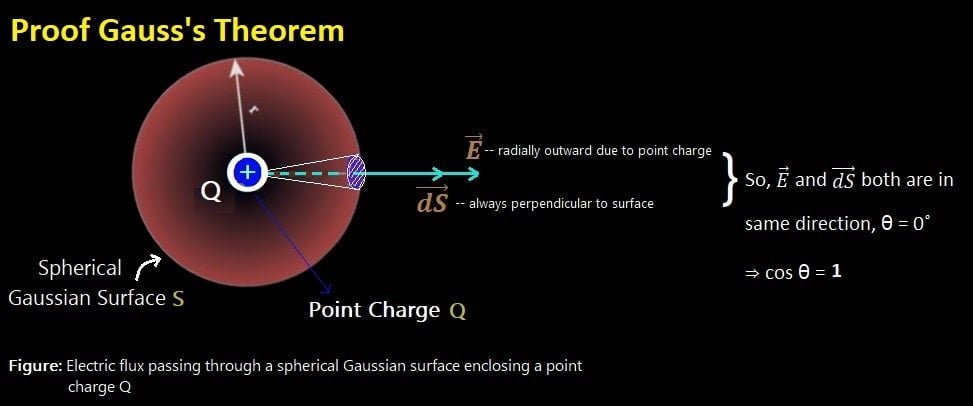
To prove Gauss Theorem we take a point charge Q kept in space. Draw an imaginary Gaussian surface symmetrically about charge distribution/point charge, which will be spherical in shape. Take a small area element dS at r distance from point charge, on Gaussian surface and find electric flux (dΦ) passing through it.

dΦ = E · dS cosθ

dΦ = E · dS ( Since, E and ds both are in same direction, so θ = 0˚ ⇒ cos θ = 1

So, total electric flux passing through spherical Gaussian surface Φ = ∮E · dS = E . ∮dS = E . S (since electric field is constant here)

Φ = E . S ………… eqⁿ (1)

Using Coulomb’s Law, the electric field intensity at any point on the Gaussian surface,

Total area of spherical Gaussian surface S = ∮dS = 4 π r²

putting value of E and S in eqⁿ (1),

Φ = E . S =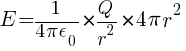

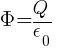
i.e. Total electric flux passing through a closed surface is equal to 1/ϵ₀ times the net charge (Q) enclosed by that surface.

Proved

👉🖱 What is Electric Flux ? Class 12 Important Topic

👉🖱 Semiconductor Electronics materials devices and simple circuits 12th Important Topic

Steps to apply Gauss’s Theorem in solving a problem:

Step 1: Define a Gaussian Surface: First, we must define a Gaussian surface that encloses the charge of interest. A Gaussian surface is an imaginary close surface drawn around charge distribution in symmetric way.

Step 2: Calculate the Electric Flux: Take a small area element ‘dS’ on Gaussian surface, draw area vector and represent direction of electric field. Now using formula Φ = ∮E · dS = E . ∮dS cosθ , calculate the electric flux passing through the Gaussian surface.

Step 3: Apply Gauss’s Law: Find value of Q_enclosed and apply Gauss’s Law, Φ = (Q_enclosed) / ϵ₀

Step 4: Evaluate the Electric Field: Compare equations obtained in step-2 and step-3, find the electric field at a point on the Gaussian surface.

Frequently Asked Questions (FAQs) from Gauss Theorem

A point charge is placed at the centre of a spherical Gaussian surface. How will the electric flux change if the sphere is replaced by a cube of the same or different volume?

Answer: Electric flux will not change because it depends only on the total charge enclosed by the Gaussian surface, according to Gauss’s theorem.

A point charge is placed at the centre of a spherical Gaussian surface. How will the electric flux change if a second charge is placed near and outside the original sphere?

Answer: Electric flux will not change because, according to Gauss’s theorem, total flux is determined by the charge inside the surface and not on the outside.

A point charge is placed at the centre of a spherical Gaussian surface. How will the electric flux change if a second charge is placed inside the sphere?

Answer: Electric flux will change because, according to Gauss’s theorem, total flux is determined by the charge inside the Gaussian surface and has been changed.

A point charge is placed at the centre of a spherical Gaussian surface. How will the electric flux change if the original charge is replaced by an electric dipole?

Answer: Electric flux will become zero because the net charge on a dipole is zero Q_enclosed = 0, so Φ = 0 .

What is the significance of Gauss’s Theorem in electrostatics?

Answer: Gauss’s Theorem in electrostatics is a powerful tool for finding electric fields due to symetric charge distributions. This theorem simplifies complex calculations and enables us to calculate the electric flux passing through closed surfaces and relate it to the enclosed charge.

What is the physical significance of electric flux?

Answer: Electric flux represents the total number of electric field lines passing through a surface. It provides information about the electric fields and the distribution of charges. High electric flux indicates a strong electric field, while low flux corresponds to a weaker field.

How is Gauss’s Theorem applied in practical applications?

Gauss’s Theorem finds numerous applications in various fields, including electrical engineering, physics, and telecommunications. It is used to analyze the behavior of electric fields, design electrical systems, calculate capacitance, and understand the properties of conductors and insulators.

Can Gauss’s Theorem be extended to other physical phenomena?

While Gauss’s Theorem is primarily applied in electrostatics, similar principles can be extended to other physical phenomena involving fields. For example, Gauss’s Law for magnetism relates the magnetic flux passing through a closed surface to the magnetic field and the net magnetic charge enclosed. This demonstrates the versatility and broad applicability of Gauss’s Theorem.

MY YouTube Channel Link 👉🖱 : https://www.youtube.com/channel/UCGpC7nWE0-bBv9I53MM8qjQ

4 basic Conservation Law Additive nature of charge Applications of concept of Accuracy and Precision Applications of Dimensional Analysis Atoms and Nuclei Test Paper class 5 hindi Class 5 Maths Class 10 Physics Combination of Error Conservation of charge Constant Error Coulomb's law de-Broglie’s equation Detection and measurement of charge: Gold-leaf electroscope Determine the value of the Planck's constant Difference between Accuracy and Precision Dual Nature of Matter Dual Nature of Radiation Ecological Pyramid Einstein’s Photoelectric Equation Electric Charge Electric field intensity Electromagnetic Spectrum Electron Emission Electrostatics EMI and AC EM Waves Test Paper Examples of Force in Daily Life Force and Laws of Motion QUIZ Fundamental Forces in Nature Graph between momentum and velocity Hertz’s Experiment Hindi grammar Hindi Vyakaran Notes Inertia Instrumental Error Law of Inertia Least Count Error Levels of Organization in Ecology Limitations of Coulomb's law limitations of dimensional analysis Linear charge density Linear Charge Distribution : Electrostatics Measurement of Errors Momentum Nitrogen Fixation Physics Principle of Homogeneity Properties of Electric Charge Quantization of charge quiz Reductionism Relation between Kinetic energy and momentum RO/ARO Hindi Vyakaran Syllabus SI unit of electric field intensity Surface Charge density Systematic Errors Transverse Nature of Electromagnetic Waves Types of Error Unification UP Special GK Volume charge density Work Function पर्यावरण और पारिस्थितिकी पर्यावरण के घटक (Components of Environment) मेरी शिक्षा

State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem,

State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem, State and Prove Gauss Theorem