Electrostatics Short answer type Questions
Electrostatics Short answer type Questions
Draw schematically an equipotential surface of a (a) uniform electrostatic field along x-axis (b) positive point charge.
Sketch electric field lines and equipotential surface due to (a) two equal positive charges near each other (b) an electric dipole.
Name the physical quantity whose SI unit is volt/meter. Is it a scalar or a vector quantity ?
Ans. Electric field intensity. It is a vector quantity.
Two point charges repel each other with a force F when placed in water of dielectric constant 81. What will the force between them when placed the same distance apart in air ?
Ans: Given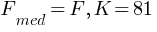 
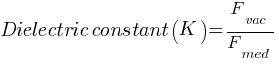
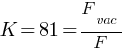
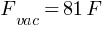
Electric dipole moment of CuSO4 molecule is 3.2 × 10-32 Cm. Find the separation between copper and sulphate ions.
Ans. Dipole moment (p) = q × (2a) , Charge on copper and sulphate ions = 2e = 2 × 1.6 × 10-19 C = 3.2 × 10-19 C
p = 3.2 × 10-32 Cm = q × (2a)
Separation between copper and sulphate ions (2a) = p / q = 3.2 × 10-32 Cm / 3.2 × 10-19 C = 10-13 m
Net capacitance of three identical capacitors connected in parallel is 12 microfarad. What will be the net capacitance when two of them are connected in (i) parallel (ii) series ?
Ans: When 3 capacitors are connected in parallel combination, Ceq = C1 + C2 + C3
Since, capacitors are identical (C1 = C2 = C3 = C) so, Ceq = 3C = 12 μF ➡️ C = 4 μF
(i) Net capacitance = ? When two of them are connected in parallel Cparallel = C1 + C2
Cparallel = C1 + C2 = 4 + 4 = 8 μF
(ii) Net capacitance = ? When two of them are connected in series
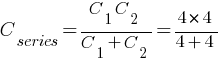= 2 μF
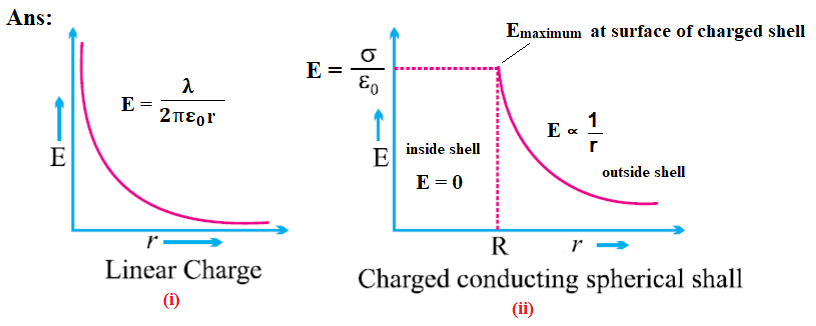
Draw the electric field Vs distance (from the centre) graph for (i) a long charged rod having linear charge density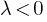 
(ii) spherical shell of radius R and charge Q > 0.
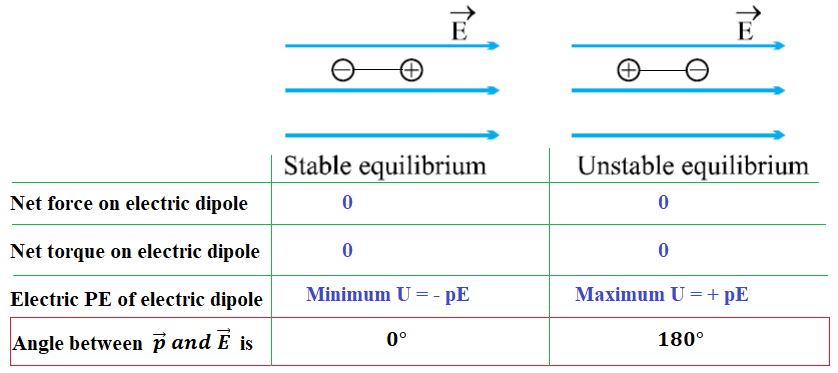
Diagrammatically represent the position of a dipole in (i) stable (ii) unstable equilibrium when placed in a uniform electric field.
A charge Q is distribution over a metal sphere of radius R. What is the electric field and electric potential at the centre ?
Ans: Electric field at centre of a charged metallic sphere, E = 0. Because charge inside conducting sphere is zero.
Electric potential at the centre of a charged metallic sphere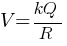 
If a body contains n1 electrons and n2 protons then what is the total charge on the body ?
Ans: Q = q1 + q2 +…. + qn. (Additive property of charge)
Q = (n2 – n1)e
How does the energy of dipole change when it is rotated from unstable equilibrium to stable equilibrium in a uniform electric field.
Ans: Work done W = – pE (cosθ2 – cosθ1 )
θ1 = Initial angle between p and E = 180° (Given: under unstable equilibrium)
θ2 = Final angle between p and E = 0° (Given: under stable equilibrium)
putting value of θ1 and θ2 in above formula,
W = – pE {cos 0° – cos 180°} = – pE {1 – (-1)} = +2pE = 2pE i.e., energy decreases.